What is the Schrödinger equation? how it’s used

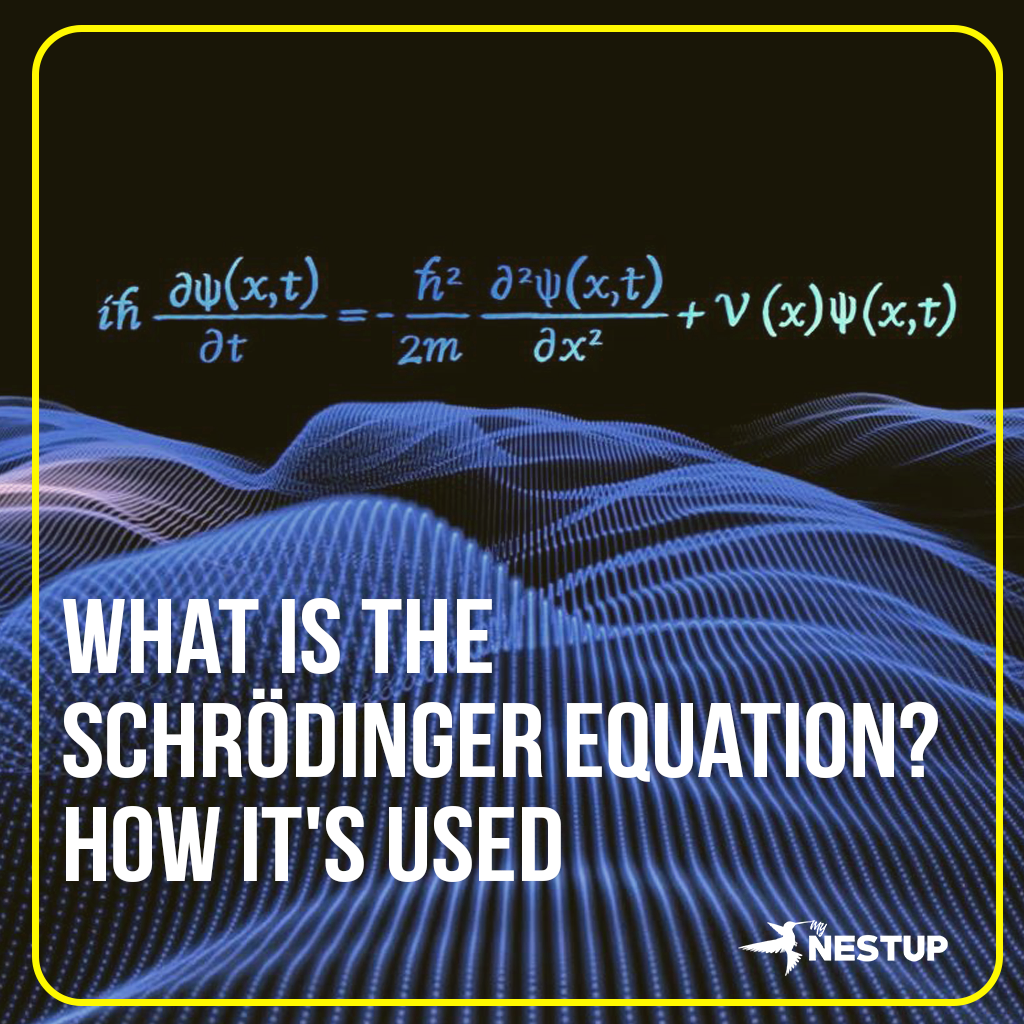
Schrödinger equation is a partial differential equation that describes how the wave function of a quantum system evolves over time. The wave function (Ψ) is a mathematical description of the state of a quantum particle. It provides information about the probabilities of finding the particle in different locations.

How the Schrödinger equation is expressed

iℏ ∂Ψ/∂t = HΨ

where i is the imaginary unit, ℏ is the reduced Planck constant, t is time, and H is the Hamiltonian operator, which is a mathematical object that describes the total energy of the system.

Schrödinger’s equation is a central equation in quantum mechanics. It is used to determine the time evolution of the wave function of a quantum system. By solving Schrödinger’s equation, we can determine the wave function of a quantum system. The determined wave function helps in calculating the probabilities of finding the particle in different states.

Schrödinger’s equation is a linear equation, which means that two wave functions are solutions to the equation. This property leads to the concept of superposition in quantum mechanics. where a particle can exist in multiple states simultaneously.

The practical applications in physics and chemistry include:

1. Quantum mechanics: Schrödinger’s equation is the fundamental equation of quantum mechanics, describing the time evolution of a quantum system.
2. Atom and molecule structure: Schrödinger’s equation can be used to calculate the energy levels and wave functions of atoms and molecules, which are crucial for understanding their structure and chemical properties.
3. Materials science: Schrödinger’s equation can be used to predict the electronic and optical properties of materials, which is important for developing new materials for electronics, photonics, and other applications.
4. Condensed matter physics: Schrödinger’s equation can be used to study the properties of solids and liquids, including their electrical, thermal, and mechanical properties.
5. Nanotechnology: Schrödinger’s equation is important for understanding the behavior of quantum systems at the nanoscale, which is crucial for developing new nanotechnologies.

Concluding all facts Schrödinger’s equation is a central equation in many areas of physics and chemistry, with a wide range of practical applications in areas such as materials science, nanotechnology, and quantum computing.