Unlocking the Mysteries of the Hamiltonian Operator

Introduction of the Hamiltonian operator

The Hamiltonian operator (H) in quantum mechanics is a mathematical object that describes the total energy of a quantum system. It acts on the wave function of the system (Ψ) to give the time evolution of the system’s energy, as described by

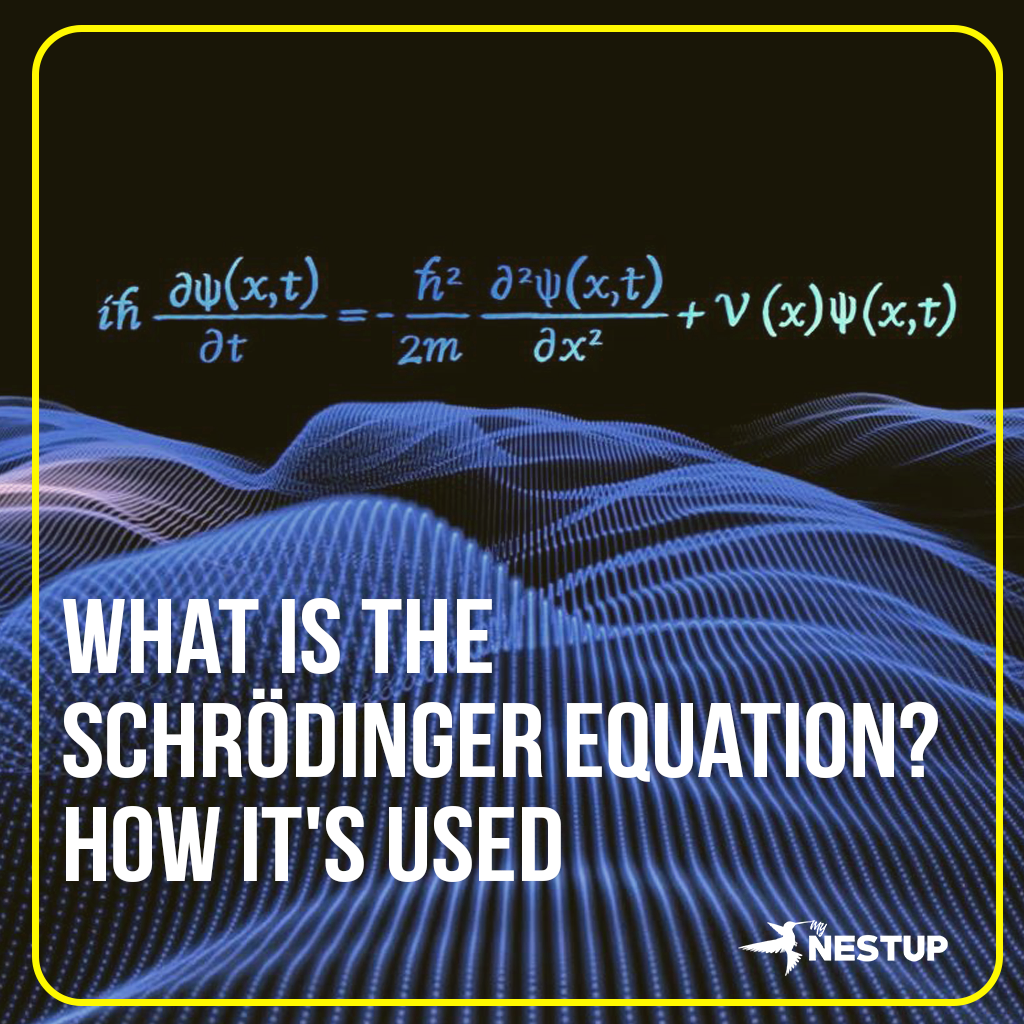
Schrödinger’s equation:

iℏ ∂Ψ/∂t = HΨ

The Hamiltonian operator can be thought of as a matrix that acts on the wave function and contains information about the potential energy and kinetic energy of the particles in the system.

In general, the Hamiltonian operator for a system can be written as a sum of several terms, each representing a different energy contribution to the system. For example, for a particle in a potential field, the

The hamiltonian operator can be written as:

H = -ℏ^2/(2m) ∇^2 + V(x, y, z)

where m is the mass of the particle, V(x, y, z) is the potential energy, and ∇^2 is the Laplacian operator, which describes the spatial derivatives of the wave function.

By solving Schrödinger’s equation, using the Hamiltonian operator, one can determine the wave function of a quantum system, and from the wave function, one can calculate the probabilities of finding the particle in different states. The Hamiltonian operator is therefore a crucial part of quantum mechanics, as it provides a complete description of the energy of a quantum system.

So here are some interesting facts about the Hamiltonian operator in quantum
mechanics:

1. The Hamiltonian operator is a fundamental concept in quantum mechanics, representing the total energy of a system. It is denoted by the symbol H and is defined as the sum of the kinetic and potential energies of all particles in the system.
2. The Hamiltonian operator is a linear operator, which means that it satisfies the superposition principle. This allows us to construct the wavefunction of a system by adding together the solutions to the time-independent Schrödinger equation for each energy level.
3. In quantum mechanics, the Hamiltonian operator is used to describe the time evolution of a system. Specifically, it determines the rate of change of the wavefunction with respect to time through the time-dependent Schrödinger equation.
4. The Hamiltonian operator is Hermitian, meaning that it is equal to its own conjugate transpose. This property is important because it ensures that the eigenvalues of the operator are real and that the eigenvectors are orthogonal.
5. The Hamiltonian operator plays a key role in determining the allowed energy levels and corresponding wavefunctions of a system. The eigenvalues of the Hamiltonian operator represent the energy levels of the system, while the corresponding eigenvectors represent the wavefunctions.
6. The Hamiltonian operator is used in a variety of applications in quantum mechanics, including in the study of atoms, molecules, and solid-state systems. It is also used in quantum field theory to describe the behavior of elementary particles and their interactions.
7. The Hamiltonian operator can be written in different forms depending on the specific physical system being studied. For example, in the case of a system of non-interacting particles, the Hamiltonian operator can be written as the sum of the individual particle Hamiltonians.
8. In quantum mechanics, the Hamiltonian operator is closely related to the uncertainty principle. Specifically, the time-energy uncertainty principle states that the uncertainty in the energy of a system is proportional to the time over which the energy is measured, as determined by the Hamiltonian operator.